Benchmarks
See:
- Matsui, H., et al. (2016), Performance benchmarks for a next generation numerical dynamo model, Geochem. Geophys. Geosyst., 17, 1586–1607, doi:10.1002/2015GC006159.
Background
The purpose of this benchmark test is to assess numerical geodynamo models on a massively parallel computational platform. We also want to encourage the testing of local methods, such as finite volume or finite element methods. We propose two kinds of benchmark tests. The first is an accuracy benchmark with the goal of establishing the resolution needed to reproduce a known numerical solution within a 1% error tolerance. The second test is a performance benchmark, which will be run using an allocation on XSEDE Stampede. We encourage participants to run accuracy benchmark tests using two different choices of magnetic boundary conditions (i.e. an insulated boundary and a pseudo vacuum boundary). However, participants are welcome to contribute results on both benchmarks using only one choice of boundary condition. For further details please see the documents below.
If you have any questions regarding this benchmark effort please contact Hiro Matsui.
Insulated Magnetic Boundary Benchmark Example
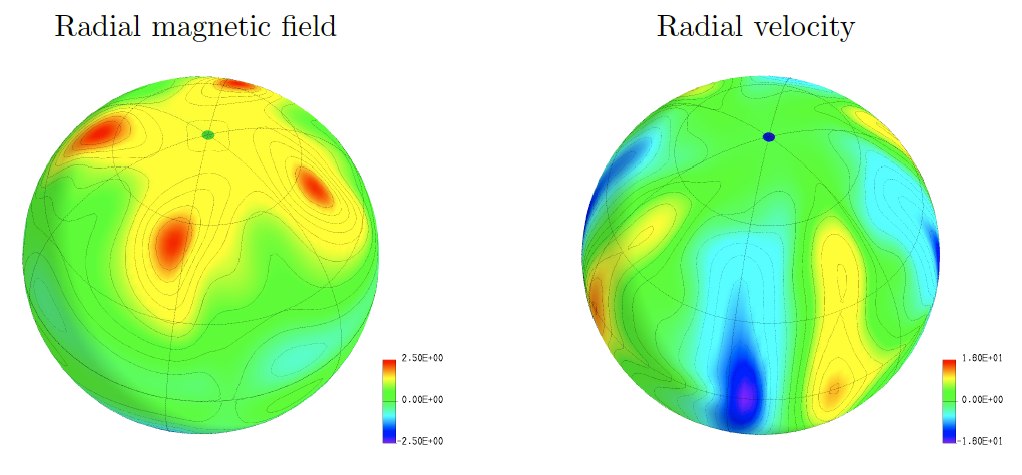 |
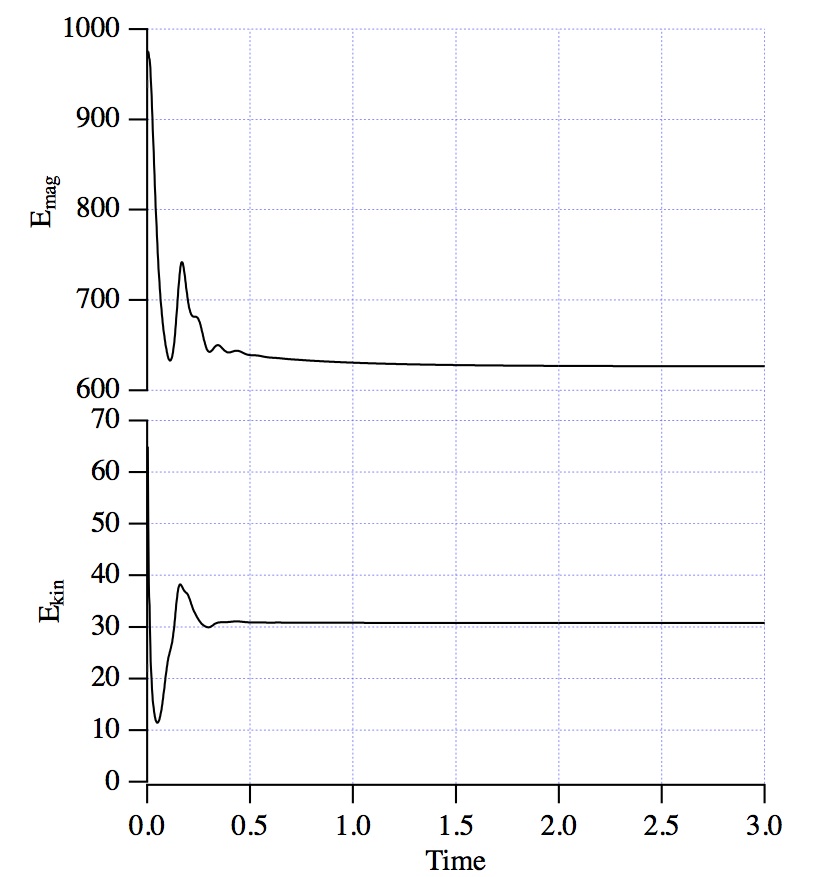 |
| Snapshot of the radial magnetic field and radial velocity. Radial magnetic field B_r at CMB r= r_o = 20/13 (left) and radial velocity u_r (right) at mid-depth of the shell in quasi-steady state for insulated magnetic boundary case. Step of contour lines for B_r and u_r are 0.25 and 2.0, respectively. | Time evolution of magnetic energy E_mag (top) and kinetic energy E_kin (bottom) for insulated magnetic boundary case to t = 3.0. |
Pseudo Vacuum Boundary Benchmark Example
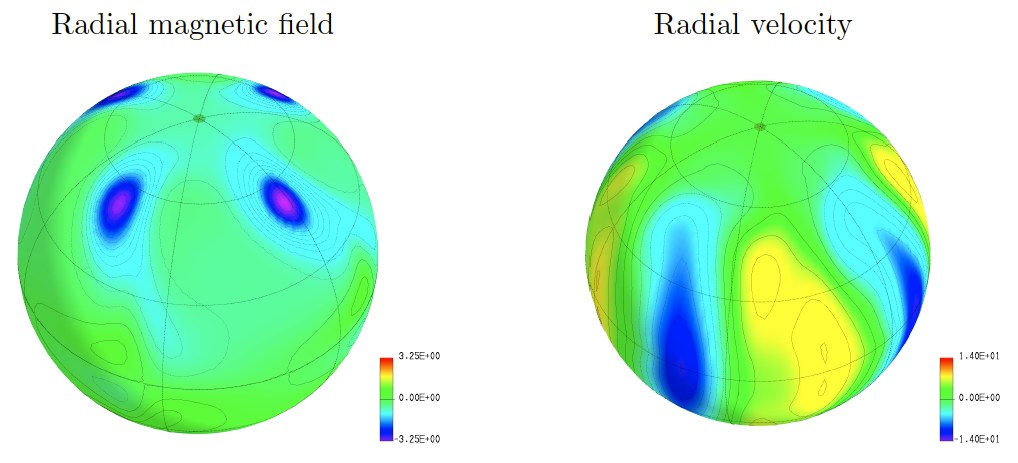 |
 |
| Snapshot of the radial magnetic field and radial velocity. Radial magnetic field B_r at CMB r= r_o = 20/13 (left) and radial velocity u_r (right) at mid-depth of the shell in quasi-steady state for pseudo vacuum boundary case. Step of contour lines for B_r and u_r are 0.25 and 2.0, respectively. | Time evolution of magnetic energy E_mag (top) and kinetic energy E_kin (bottom) for pseudo vacuum boundary case to t = 3.0. |
See also:

